Chemisty Unit 9 Worksheet 1 Fases Again Unit 7 Lecture 4 Ideal Gas Law and Daltons Law

Chapter nine. Gases

9.3 Stoichiometry of Gaseous Substances, Mixtures, and Reactions

Learning Objectives

By the finish of this department, you will be able to:

• Apply the ideal gas constabulary to compute gas densities and molar masses
• Perform stoichiometric calculations involving gaseous substances
• Land Dalton's constabulary of partial pressures and use it in calculations involving gaseous mixtures

The report of the chemical behavior of gases was part of the ground of perhaps the near central chemic revolution in history. French nobleman Antoine Lavoisier, widely regarded as the "father of modern chemistry," inverse chemistry from a qualitative to a quantitative science through his piece of work with gases. He discovered the police of conservation of thing, discovered the part of oxygen in combustion reactions, adamant the composition of air, explained respiration in terms of chemic reactions, and more. He was a casualty of the French Revolution, guillotined in 1794. Of his death, mathematician and astronomer Joseph-Louis Lagrange said, "Information technology took the mob only a moment to remove his head; a century will not suffice to reproduce it."^[1]

Every bit described in an earlier chapter of this text, nosotros tin can turn to chemical stoichiometry for answers to many of the questions that ask "How much?" Nosotros can answer the question with masses of substances or volumes of solutions. However, nosotros can as well answer this question another style: with volumes of gases. Nosotros can use the ideal gas equation to chronicle the force per unit area, volume, temperature, and number of moles of a gas. Here nosotros will combine the ideal gas equation with other equations to find gas density and molar mass. We volition bargain with mixtures of different gases, and calculate amounts of substances in reactions involving gases. This section will not introduce whatsoever new material or ideas, but will provide examples of applications and ways to integrate concepts we accept already discussed.

Density of a Gas

Think that the density of a gas is its mass to book ratio, [latex]\rho = \frac{chiliad}{V}[/latex]. Therefore, if we can decide the mass of some book of a gas, we will get its density. The density of an unknown gas can used to determine its tooth mass and thereby assist in its identification. The ideal gas police, PV = nRT, provides us with a ways of deriving such a mathematical formula to chronicle the density of a gas to its volume in the proof shown in Case one.

Example 1

Derivation of a Density Formula from the Ideal Gas Constabulary
Apply PV = nRT to derive a formula for the density of gas in g/L

Solution

1. PV = nRT
2. Rearrange to get (mol/50): [latex]\frac{n}{v} = \frac{P}{RT}[/latex]
3. Multiply each side of the equation past the molar mass, [latex]\mathcal{M}[/latex]. When moles are multiplied by [latex]\mathcal{K}[/latex] in g/mol, g are obtained:
   [latex](\mathcal{One thousand})(\frac{n}{Five}) = (\frac{P}{RT})(\mathcal{M})[/latex]
4. [latex]g \text{/Fifty} = \rho = \frac{P \mathcal{M}}{RT}[/latex]

Check Your Learning
A gas was plant to take a density of 0.0847 g/L at 17.0 °C and a pressure of 760 torr. What is its molar mass? What is the gas?

Answer:

[latex]\rho = \frac{P \mathcal{M}}{RT}[/latex]

[latex]0.0847 \;\text{grand/L} = 760 \;\rule[0.5ex]{1.7em}{0.1ex}\hspace{-1.7em}\text{torr} \times \frac{one \;\rule[0.25ex]{1.2em}{0.1ex}\hspace{-1.2em}\text{atm}}{760 \;\dominion[0.25ex]{one.2em}{0.1ex}\hspace{-1.2em}\text{torr}} \times \frac{\mathcal{Thou}}{0.0821 \;\text{L} \;\rule[0.25ex]{i.2em}{0.1ex}\hspace{-1.2em}\text{atm/mol K}} \times 290 \;\text{Thou}[/latex]

[latex]\mathcal{M}[/latex] = 2.02 g/mol; therefore, the gas must be hydrogen (H₂, 2.02 g/mol)

We must specify both the temperature and the pressure of a gas when calculating its density because the number of moles of a gas (and thus the mass of the gas) in a liter changes with temperature or pressure. Gas densities are often reported at STP.

Example 2

Empirical/Molecular Formula Problems Using the Ideal Gas Law and Density of a Gas
Cyclopropane, a gas once used with oxygen every bit a full general coldhearted, is equanimous of 85.7% carbon and 14.3% hydrogen by mass. Find the empirical formula. If 1.56 g of cyclopropane occupies a volume of 1.00 L at 0.984 atm and l °C, what is the molecular formula for cyclopropane?

Solution
Strategy: Showtime solve the empirical formula problem using methods discussed before. Assume 100 1000 and convert the per centum of each element into grams. Determine the number of moles of carbon and hydrogen in the 100-g sample of cyclopropane. Divide by the smallest number of moles to relate the number of moles of carbon to the number of moles of hydrogen. In the last step, realize that the smallest whole number ratio is the empirical formula:

[latex]\brainstorm{assortment}{fifty 50}85.7 \;\text{m C} \times \frac{1 \;\text{mol C}}{12.01 \;\text{grand C}} = 7.136 \;\text{mol C} & \frac{vii.136}{7.136} = 1.00 \;\text{mol C} \\[1em] 14.3 \;\text{g H} \times \frac{1 \;\text{mol H}}{one.01 \;\text{g H}} = 14.158 \;\text{mol H} & \frac{14.158}{7.136} = 1.98 \;\text{mol H} \end{array}[/latex]

Empirical formula is CH₂ [empirical mass (EM) of 14.03 g/empirical unit].

Next, use the density equation related to the ideal gas law to decide the molar mass:

[latex]\text{d} = \frac{P \mathcal{Thousand}}{RT} \;\;\;\;\; \frac{1.56 \;\text{chiliad}}{1.00 \;\text{L}} = 0.984 \;\text{atm} \times \frac{\mathcal{G}}{0.0821 \;\text{Fifty atm/mol K}} \times 323 \;\text{One thousand}[/latex]

[latex]\mathcal{M}[/latex] = 42.0 k/mol, [latex]\frac{\mathcal{M}}{\text{Eastward} \mathcal{1000}} = \frac{42.0}{xiv.03} = 2.99[/latex], so (three)(CH₂) = C₃H_six (molecular formula)

Check Your LearningAcetylene, a fuel used welding torches, is comprised of 92.3% C and seven.seven% H by mass. Observe the empirical formula. If ane.10 g of acetylene occupies of book of 1.00 L at 1.15 atm and 59.five °C, what is the molecular formula for acetylene?

Reply:

Empirical formula, CH; Molecular formula, C₂H₂

Molar Mass of a Gas

Another useful awarding of the platonic gas law involves the determination of molar mass. Past definition, the molar mass of a substance is the ratio of its mass in grams, m, to its corporeality in moles, north:

[latex]\mathcal{One thousand} = \frac{\text{grams of substance}}{\text{moles of substance}} = \frac{m}{n}[/latex]

The ideal gas equation can be rearranged to isolate northward:

[latex]due north = \frac{PV}{RT}[/latex]

and then combined with the tooth mass equation to yield:

[latex]\mathcal{Grand} = \frac{mRT}{PV}[/latex]

This equation can be used to derive the molar mass of a gas from measurements of its pressure, volume, temperature, and mass.

Case 3

Determining the Tooth Mass of a Volatile Liquid
The judge molar mass of a volatile liquid tin be determined by:

1. Heating a sample of the liquid in a flask with a tiny hole at the top, which converts the liquid into gas that may escape through the hole
2. Removing the flask from estrus at the instant when the last flake of liquid becomes gas, at which time the flask will be filled with only gaseous sample at ambience pressure
3. Sealing the flask and permitting the gaseous sample to condense to liquid, and so weighing the flask to decide the sample's mass (see Figure 1)

Figure 1. When the volatile liquid in the flask is heated by its boiling betoken, it becomes gas and drives air out of the flask. At t_fifty⟶g, the flask is filled with volatile liquid gas at the same pressure as the atmosphere. If the flask is then cooled to room temperature, the gas condenses and the mass of the gas that filled the flask, and is now liquid, can be measured. (credit: modification of work by Mark Ott)

Using this process, a sample of chloroform gas weighing 0.494 g is collected in a flask with a book of 129 cm³ at 99.6 °C when the atmospheric pressure is 742.1 mm Hg. What is the estimate molar mass of chloroform?

Solution
Since [latex]\mathcal{Grand} = \frac{m}{northward}[/latex] and [latex]due north = \frac{PV}{RT}[/latex], substituting and rearranging gives [latex]\mathcal{K} = \frac{mRT}{PV}[/latex],

then

[latex]\mathcal{M} = \frac{mRT}{PV} = \frac{(0.494 \;\text{g}) \times 0.08206 \;\text{Fifty} \cdot \text{atm/mol Grand} \times 372.8 \;\text{K}}{0.976 \;\text{atm} \times \; 0.129 \;\text{Fifty}} = 120 \;\text{g/mol}[/latex]

Check Your Learning
A sample of phosphorus that weighs 3.243 × 10⁻² grand exerts a pressure of 31.89 kPa in a 56.0-mL bulb at 550 °C. What are the molar mass and molecular formula of phosphorus vapor?

The Force per unit area of a Mixture of Gases: Dalton's Constabulary

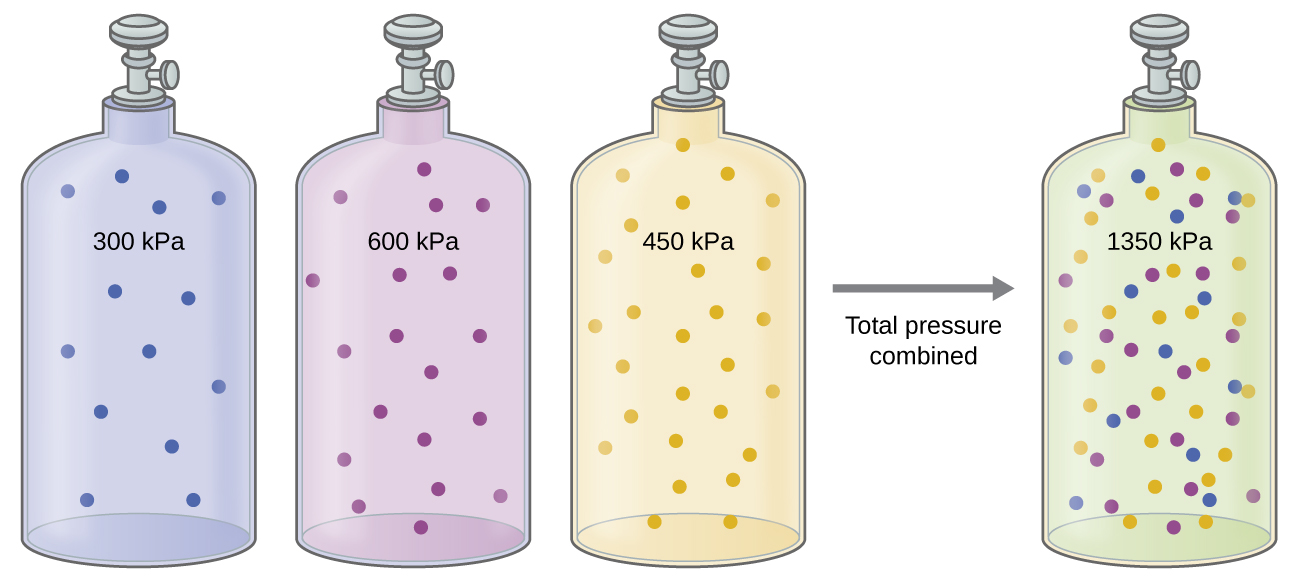
Unless they chemically react with each other, the individual gases in a mixture of gases do not affect each other'due south pressure. Each individual gas in a mixture exerts the same pressure that information technology would exert if it were present lone in the container (Figure 2). The pressure exerted past each individual gas in a mixture is called its partial pressure. This observation is summarized by Dalton'southward police of fractional pressures: The full pressure level of a mixture of ideal gases is equal to the sum of the partial pressures of the component gases:

[latex]P_{Full} = P_A + P_B + P_C + \cdots = \sum_{\text{i}} P_\text{i}[/latex]

In the equation P_Total is the total pressure of a mixture of gases, P_A is the partial pressure level of gas A; P_B is the partial pressure of gas B; P_C is the partial pressure of gas C; and and so on.

Figure 2. If equal-book cylinders containing gas A at a pressure of 300 kPa, gas B at a pressure of 600 kPa, and gas C at a pressure of 450 kPa are all combined in the aforementioned-size cylinder, the full pressure of the mixture is 1350 kPa.

The fractional pressure of gas A is related to the total pressure of the gas mixture via its mole fraction (X), a unit of concentration defined as the number of moles of a component of a solution divided past the total number of moles of all components:

[latex]P_A = X_A \times P_{Full} \;\;\;\;\; \text{where} \;\;\;\;\; X_A = \frac{n_A}{n_{Total}}[/latex]

where P_A , X_A , and n_A are the fractional pressure, mole fraction, and number of moles of gas A, respectively, and n_Full is the number of moles of all components in the mixture.

Example 4

The Pressure of a Mixture of Gases
A x.0-L vessel contains two.l × 10⁻³ mol of H₂, 1.00 × ten⁻ⁱⁱⁱ mol of He, and 3.00 × x⁻⁴ mol of Ne at 35 °C.

(a) What are the partial pressures of each of the gases?

(b) What is the total pressure level in atmospheres?

Solution
The gases deport independently, and then the fractional pressure of each gas tin can exist adamant from the ideal gas equation, using [latex]P = \frac{nRT}{V}[/latex]:

[latex]P_{\text{H}_2} = \frac{(two.50 \times 10^{-three} \;\rule[0.5ex]{1.2em}{0.1ex}\hspace{-one.2em}\text{mol})(0.08206 \;\rule[0.5ex]{0.5em}{0.1ex}\hspace{-0.5em}\text{50} \;\text{atm} \;\rule[0.5ex]{iii.5em}{0.1ex}\hspace{-3.5em}\text{mol}^{-1} \text{Thou}^{-one})(308 \;\dominion[0.5ex]{0.5em}{0.1ex}\hspace{-0.5em}\text{K})}{ten.0 \;\rule[0.5ex]{0.5em}{0.1ex}\hspace{-0.5em}\text{Fifty}} = half dozen.32 \times x^{-3} \;\text{atm}[/latex]

[latex]P_\text{He} = \frac{(1.00 \times 10^{-3} \;\dominion[0.5ex]{1.2em}{0.1ex}\hspace{-1.2em}\text{mol})(0.08206 \;\rule[0.5ex]{0.5em}{0.1ex}\hspace{-0.5em}\text{Fifty} \;\text{atm} \;\dominion[0.5ex]{3.5em}{0.1ex}\hspace{-3.5em}\text{mol}^{-ane} \text{K}^{-1})(308 \;\rule[0.5ex]{0.5em}{0.1ex}\hspace{-0.5em}\text{K})}{ten.0 \;\rule[0.5ex]{0.5em}{0.1ex}\hspace{-0.5em}\text{50}} = ii.53 \times 10^{-3} \;\text{atm}[/latex]

[latex]P_\text{Ne} = \frac{(3.00 \times ten^{-4} \;\dominion[0.5ex]{1.2em}{0.1ex}\hspace{-1.2em}\text{mol})(0.08206 \;\rule[0.5ex]{0.5em}{0.1ex}\hspace{-0.5em}\text{Fifty} \;\text{atm} \;\dominion[0.5ex]{3.5em}{0.1ex}\hspace{-3.5em}\text{mol}^{-ane} \text{G}^{-1})(308 \;\rule[0.5ex]{0.5em}{0.1ex}\hspace{-0.5em}\text{K})}{10.0 \;\rule[0.5ex]{0.5em}{0.1ex}\hspace{-0.5em}\text{L}} = vii.58 \times 10^{-iv} \;\text{atm}[/latex]

The total force per unit area is given by the sum of the fractional pressures:

[latex]P_\text{T} = P_{\text{H}_2} + P_\text{He} + P_\text{Ne} = (0.00632 + 0.00253 + 0.00076) \;\text{atm} = 9.61 \times x^{-3} \;\text{atm}[/latex]

Bank check Your Learning
A v.73-L flask at 25 °C contains 0.0388 mol of Due north₂, 0.147 mol of CO, and 0.0803 mol of H₂. What is the total force per unit area in the flask in atmospheres?

Here is some other example of this concept, but dealing with mole fraction calculations.

Case 5

The Pressure of a Mixture of Gases
A gas mixture used for anesthesia contains 2.83 mol oxygen, O₂, and eight.41 mol nitrous oxide, Northward_iiO. The total pressure of the mixture is 192 kPa.

(a) What are the mole fractions of O_two and N_twoO?

(b) What are the partial pressures of O₂ and N₂O?

Solution
The mole fraction is given by [latex]X_A = \frac{n_A}{n_{Total}}[/latex] and the fractional pressure is [latex]P_A = X_A \times P_{Total}[/latex].

For O₂,

[latex]X_{O_2} = \frac{n_{O_2}}{n_{Total}} = \frac{2.83 \;\text{mol}}{(2.83 + 8.41) \;\text{mol}} = 0.252[/latex]

and [latex]P_{O_2} = X_{O_2} \times P_{Total} = 0.252 \times 192 \;\text{kPa} = 48.iv \;\text{kPa}[/latex]

For Due north_twoO,

[latex]X_{N_2} = \frac{n_{N_2}}{n_{Total}} = \frac{8.41 \;\text{mol}}{(2.83 + 8.41) \;\text{mol}} = 0.748[/latex]

and

[latex]P_{N_2} = X_{N_2} \times P_{Total} = 0.748 \times 192 \;\text{kPa} = 143.6 \;\text{kPa}[/latex]

Check Your Learning
What is the force per unit area of a mixture of 0.200 g of H₂, one.00 one thousand of N₂, and 0.820 g of Ar in a container with a volume of 2.00 L at 20 °C?

Drove of Gases over H2o

A simple way to collect gases that practise non react with water is to capture them in a bottle that has been filled with water and inverted into a dish filled with water. The pressure level of the gas inside the bottle can be made equal to the air pressure exterior past raising or lowering the bottle. When the h2o level is the same both within and outside the bottle (Figure three), the pressure level of the gas is equal to the atmospheric pressure level, which can be measured with a barometer.

Effigy 3. When a reaction produces a gas that is collected above water, the trapped gas is a mixture of the gas produced by the reaction and h2o vapor. If the collection flask is appropriately positioned to equalize the water levels both inside and outside the flask, the pressure of the trapped gas mixture will equal the atmospheric pressure outside the flask (encounter the earlier give-and-take of manometers).

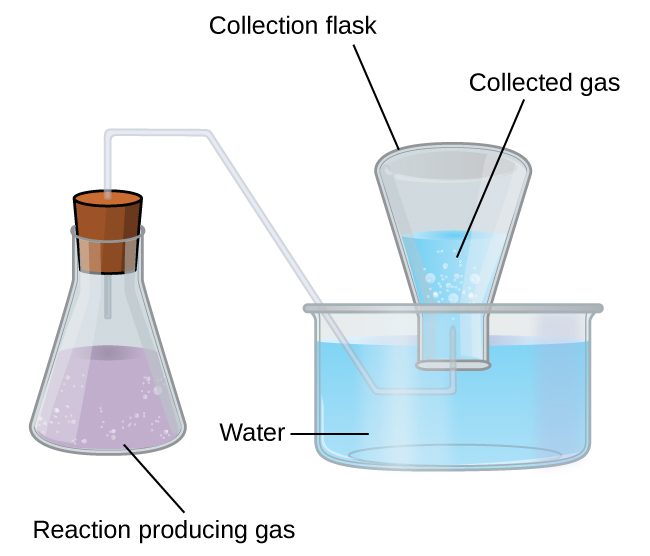
Nevertheless, in that location is some other factor we must consider when we measure out the pressure of the gas by this method. Water evaporates and there is always gaseous h2o (water vapor) in a higher place a sample of liquid h2o. As a gas is collected over h2o, it becomes saturated with h2o vapor and the total pressure level of the mixture equals the partial pressure of the gas plus the partial pressure of the water vapor. The pressure of the pure gas is therefore equal to the full pressure minus the force per unit area of the water vapor—this is referred to as the "dry" gas pressure, that is, the pressure of the gas only, without water vapor. The vapor pressure of water, which is the pressure level exerted by water vapor in equilibrium with liquid water in a closed container, depends on the temperature (Effigy 4); more detailed information on the temperature dependence of water vapor can exist establish in Table ii, and vapor pressure level will be discussed in more detail in the next chapter on liquids.

Figure 4. This graph shows the vapor pressure of water at bounding main level as a function of temperature.

Temperature (°C)	Force per unit area (torr)		Temperature (°C)	Pressure (torr)		Temperature (°C)	Pressure (torr)
–10	1.95		18	15.5		xxx	31.eight
–5	three.0		19	16.5		35	42.2
–2	3.9		twenty	17.5		40	55.3
0	four.six		21	18.7		l	92.5
ii	5.3		22	19.8		lx	149.iv
iv	half dozen.one		23	21.1		70	233.7
six	7.0		24	22.4		80	355.1
8	8.0		25	23.eight		ninety	525.8
10	9.two		26	25.two		95	633.9
12	10.5		27	26.7		99	733.two
fourteen	12.0		28	28.iii		100.0	760.0
xvi	13.6		29	30.0		101.0	787.6
Table 2. Vapor Pressure of Water ice and Water in Various Temperatures at Sea Level

Example 6

Force per unit area of a Gas Nerveless Over H2o
If 0.200 50 of argon is collected over water at a temperature of 26 °C and a pressure of 750 torr in a arrangement like that shown in Figure three, what is the partial pressure of argon?

Solution
Co-ordinate to Dalton's law, the total pressure in the canteen (750 torr) is the sum of the partial pressure of argon and the partial pressure level of gaseous h2o:

[latex]P_\text{T} = P_\text{Ar} + P_{{\text{H}_2}\text{O}}[/latex]

Rearranging this equation to solve for the pressure of argon gives:

[latex]P_\text{Ar} = P_\text{T} - P_{{\text{H}_2}\text{O}}[/latex]

The pressure of h2o vapor above a sample of liquid water at 26 °C is 25.ii torr (Appendix Eastward), then:

[latex]P_\text{Ar} = 750 \;\text{torr} - 25.2 \;\text{torr} = 725 \;\text{torr}[/latex]

Check Your Learning
A sample of oxygen nerveless over h2o at a temperature of 29.0 °C and a pressure of 764 torr has a book of 0.560 L. What volume would the dry oxygen have under the same conditions of temperature and pressure?

Chemic Stoichiometry and Gases

Chemical stoichiometry describes the quantitative relationships betwixt reactants and products in chemical reactions.

We have previously measured quantities of reactants and products using masses for solids and volumes in conjunction with the molarity for solutions; now we can also employ gas volumes to indicate quantities. If we know the volume, pressure, and temperature of a gas, nosotros can utilise the ideal gas equation to calculate how many moles of the gas are present. If nosotros know how many moles of a gas are involved, we tin calculate the book of a gas at any temperature and pressure level.

Avogadro's Law Revisited

Sometimes we can accept advantage of a simplifying feature of the stoichiometry of gases that solids and solutions do non exhibit: All gases that show ideal behavior contain the aforementioned number of molecules in the same volume (at the same temperature and pressure). Thus, the ratios of volumes of gases involved in a chemic reaction are given by the coefficients in the equation for the reaction, provided that the gas volumes are measured at the same temperature and pressure.

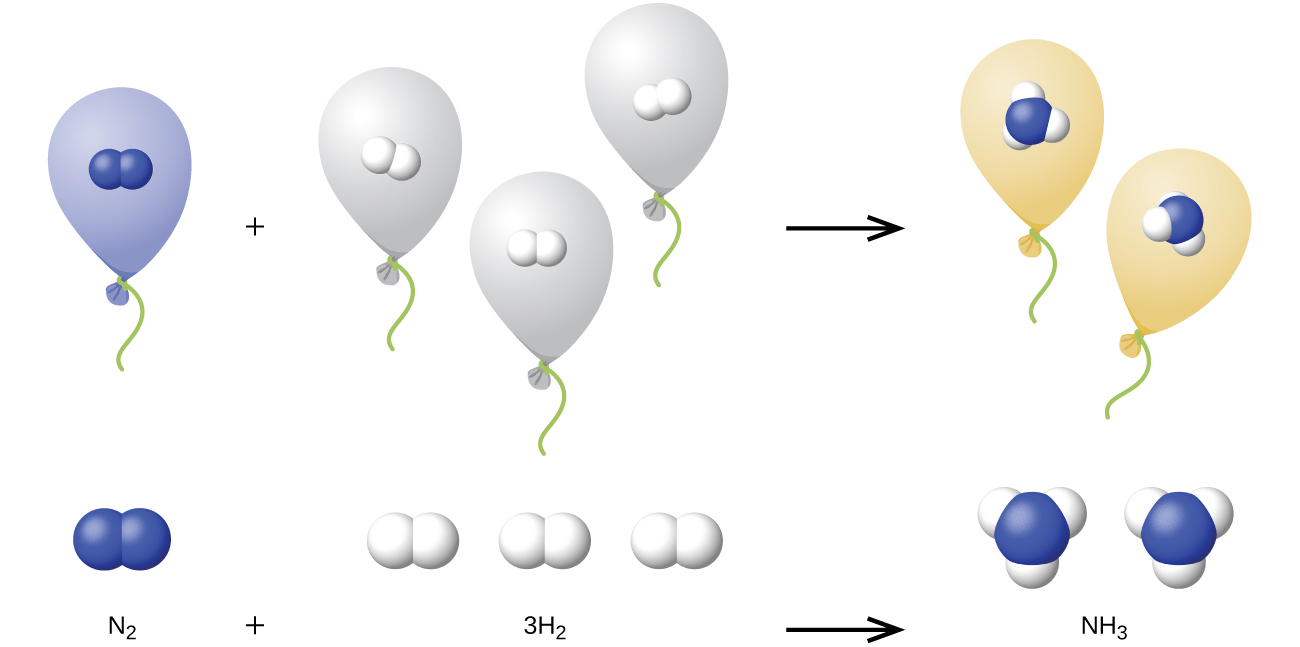
We can extend Avogadro's law (that the volume of a gas is directly proportional to the number of moles of the gas) to chemical reactions with gases: Gases combine, or react, in definite and simple proportions past volume, provided that all gas volumes are measured at the same temperature and pressure level. For example, since nitrogen and hydrogen gases react to produce ammonia gas according to [latex]\text{N}_2(g) + iii\text{H}_2(g) \longrightarrow two\text{NH}_3(g)[/latex], a given book of nitrogen gas reacts with three times that volume of hydrogen gas to produce two times that volume of ammonia gas, if pressure level and temperature remain constant.

The explanation for this is illustrated in Effigy 5. Co-ordinate to Avogadro's constabulary, equal volumes of gaseous Northward_ii, H₂, and NH₃, at the same temperature and pressure level, contain the aforementioned number of molecules. Because ane molecule of N₂ reacts with three molecules of H_ii to produce ii molecules of NH_iii, the book of H₂ required is three times the volume of Due north_two, and the volume of NH_iii produced is two times the volume of N₂.

Figure five. One volume of N₂ combines with three volumes of H₂ to form two volumes of NH_three.

Instance 7

Reaction of Gases
Propane, C₃H₈(grand), is used in gas grills to provide the heat for cooking. What volume of O_two(g) measured at 25 °C and 760 torr is required to react with two.seven L of propane measured under the same conditions of temperature and pressure? Assume that the propane undergoes consummate combustion.

Solution
The ratio of the volumes of C₃H₈ and O₂ will exist equal to the ratio of their coefficients in the balanced equation for the reaction:

[latex]\begin{array}{l c r} \text{C}_3 \text{H}_8(g) + 5\text{O}_2(g) & \longrightarrow & 3\text{CO}_2(g) + iv\text{H}_2 \text{O}(l) \\[1em] 1 \;\text{volume} + v \;\text{volumes} & & 3 \;\text{volumes} + four \;\text{volumes} \end{array}[/latex]

From the equation, we see that 1 volume of C₃H₈ volition react with 5 volumes of O₂:

[latex]2.7 \;\rule[0.75ex]{three.2em}{0.1ex}\hspace{-3.2em}\text{Fifty C}_3 \text{H}_8 \times \frac{5 \;\text{L O}_2}{1 \;\rule[0.35ex]{2.5em}{0.1ex}\hspace{-ii.5em}\text{50 C}_3 \text{H}_8} = 13.v \;\text{50 O}_2[/latex]

A volume of 13.5 L of O₂ will be required to react with 2.seven L of C_threeH₈.

Cheque Your Learning
An acetylene tank for an oxyacetylene welding torch provides 9340 50 of acetylene gas, C₂H₂, at 0 °C and i atm. How many tanks of oxygen, each providing 7.00 × 10^three L of O₂ at 0 °C and 1 atm, volition exist required to burn the acetylene?

[latex]2\text{C}_2 \text{H}_2 + 5\text{O}_2 \longrightarrow 4\text{CO}_2 + 2\text{H}_2 \text{O}[/latex]

Respond:

3.34 tanks (2.34 × 10^iv Fifty)

Instance viii

Volumes of Reacting Gases
Ammonia is an of import fertilizer and industrial chemical. Suppose that a book of 683 billion cubic feet of gaseous ammonia, measured at 25 °C and 1 atm, was manufactured. What volume of H_two(one thousand), measured under the same weather, was required to prepare this amount of ammonia by reaction with N₂?

[latex]\text{North}_2(g) + 3\text{H}_2(g) \longrightarrow ii\text{NH}_3(chiliad)[/latex]

Solution
Because equal volumes of H_ii and NH₃ contain equal numbers of molecules and each three molecules of H₂ that react produce two molecules of NH₃, the ratio of the volumes of H₂ and NH₃ will exist equal to 3:two. Two volumes of NH₃, in this instance in units of billion ftⁱⁱⁱ, will exist formed from three volumes of H₂:

[latex]683 \;\rule[0.75ex]{6.5em}{0.1ex}\hspace{-6.5em}\text{billion ft}^iii \;\text{NH}_3 \times \frac{3 \;\text{billion ft}^3 \;\text{H}_2}{2 \;\dominion[0.5ex]{4.7em}{0.1ex}\hspace{-iv.7em}\text{billion ft}^3 \;\text{NH}_3} = 1.02 \times 10^three \;\text{billion ft}^3 \;\text{H}_2[/latex]

The manufacture of 683 billion ft^three of NH_iii required 1020 billion ft³ of H₂. (At 25 °C and 1 atm, this is the volume of a cube with an edge length of approximately 1.9 miles.)

Bank check Your Learning
What volume of O₂(grand) measured at 25 °C and 760 torr is required to react with 17.0 L of ethylene, C₂H₄(g), measured nether the aforementioned weather of temperature and force per unit area? The products are CO₂ and water vapor.

Instance ix

Volume of Gaseous Product
What volume of hydrogen at 27 °C and 723 torr may exist prepared by the reaction of viii.88 1000 of gallium with an excess of muriatic acid?

[latex]ii\text{Ga}(s) + 6 \text{HCl}(aq) \longrightarrow two\text{GaCl}_3 (aq) + 3\text{H}_2(g)[/latex]

Solution
To catechumen from the mass of gallium to the book of H_ii(thou), we demand to practice something like this:

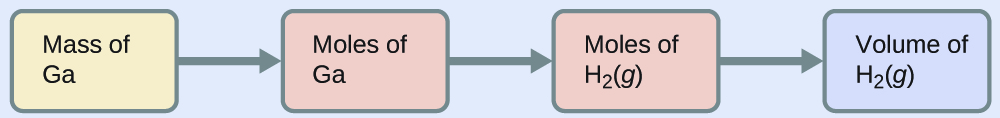
The starting time two conversions are:

[latex]8.88 \;\rule[0.75ex]{ii.5em}{0.1ex}\hspace{-2.5em}\text{m Ga} \times \frac{one \;\rule[0.5ex]{two.5em}{0.1ex}\hspace{-2.5em}\text{mol Ga}}{69.723 \;\rule[0.5ex]{i.5em}{0.1ex}\hspace{-i.5em}\text{grand Ga}} \times \frac{3 \;\text{mol H}_2}{ii \;\rule[0.5ex]{2.5em}{0.1ex}\hspace{-2.5em}\text{mol Ga}} = 0.191 \;\text{mol H}_2[/latex]

Finally, nosotros can employ the ideal gas police:

[latex]V_{\text{H}_2} = (\frac{nRT}{P})_{\text{H}_2} = \frac{0.191 \;\rule[0.5ex]{1.25em}{0.1ex}\hspace{-i.25em}\text{mol} \times 0.08206 \;\text{L} \;\rule[0.5ex]{four.5em}{0.1ex}\hspace{-4.5em}\text{atm mol}^{-1} \text{K}^{-1} \times 300 \;\text{Chiliad}}{0.951 \;\dominion[0.5ex]{1.4em}{0.1ex}\hspace{-1.4em}\text{atm}} = iv.94 \;\text{50}[/latex]

Check Your Learning
Sulfur dioxide is an intermediate in the preparation of sulfuric acid. What book of SO_two at 343 °C and 1.21 atm is produced by burning l.00 kg of sulfur in oxygen?

Greenhouse Gases and Climate change

The thin skin of our atmosphere keeps the earth from being an ice planet and makes information technology habitable. In fact, this is due to less than 0.5% of the air molecules. Of the energy from the sun that reaches the earth, almost [latex]\frac{one}{3}[/latex] is reflected dorsum into space, with the rest captivated past the atmosphere and the surface of the earth. Some of the free energy that the globe absorbs is re-emitted as infrared (IR) radiations, a portion of which passes back out through the atmosphere into space. Nevertheless, most of this IR radiation is captivated past certain substances in the temper, known equally greenhouse gases, which re-emit this energy in all directions, trapping some of the heat. This maintains favorable living weather condition—without atmosphere, the average global average temperature of 14 °C (57 °F) would be about –19 °C (–2 °F). The major greenhouse gases (GHGs) are water vapor, carbon dioxide, methane, and ozone. Since the Industrial Revolution, human activity has been increasing the concentrations of GHGs, which have inverse the energy balance and are significantly altering the earth'south climate (Figure half dozen).

Figure 6. Greenhouse gases trap plenty of the lord's day's energy to make the planet habitable—this is known every bit the greenhouse outcome. Human activities are increasing greenhouse gas levels, warming the planet and causing more extreme weather events.

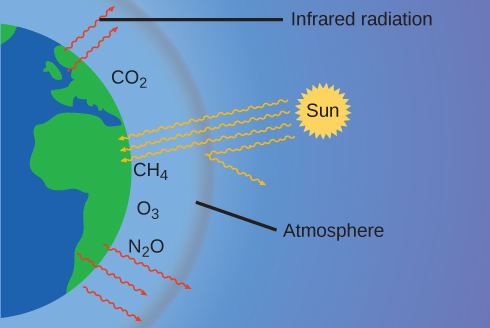
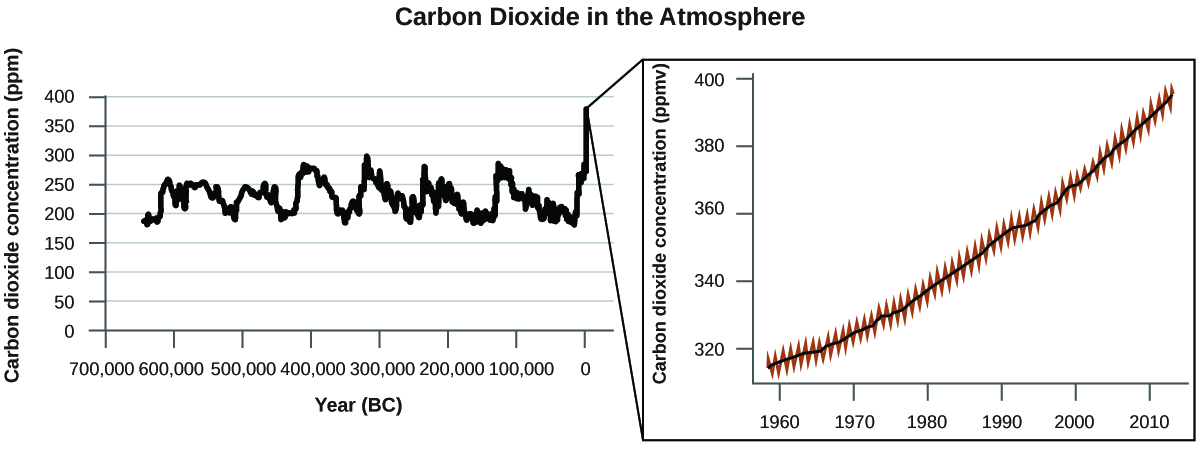
There is strong evidence from multiple sources that higher atmospheric levels of CO_two are caused by human activity, with fossil fuel called-for accounting for near [latex]\frac{3}{four}[/latex] of the contempo increase in CO₂. Reliable data from water ice cores reveals that CO₂ concentration in the atmosphere is at the highest level in the past 800,000 years; other evidence indicates that information technology may be at its highest level in 20 1000000 years. In contempo years, the CO₂ concentration has increased from historical levels of below 300 ppm to almost 400 ppm today (Figure vii).

Figure 7. CO₂ levels over the past 700,000 years were typically from 200–300 ppm, with a steep, unprecedented increase over the past l years.

Click here to see a two-minute video explaining greenhouse gases and global warming.

Susan Solomon

Atmospheric and climate scientist Susan Solomon (Figure 8) is the author of one of The New York Times books of the year (The Coldest March, 2001), one of Time magazine's 100 well-nigh influential people in the world (2008), and a working group leader of the Intergovernmental Panel on Climate Change (IPCC), which was the recipient of the 2007 Nobel Peace Prize. She helped decide and explain the cause of the formation of the ozone hole over Antarctica, and has authored many important papers on climate alter. She has been awarded the top scientific honors in the US and French republic (the National Medal of Scientific discipline and the Grande Medaille, respectively), and is a member of the National Academy of Sciences, the Royal Lodge, the French Academy of Sciences, and the European Academy of Sciences. Formerly a professor at the University of Colorado, she is at present at MIT, and continues to work at NOAA.

For more data, picket this video nearly Susan Solomon.

Figure eight. Susan Solomon's research focuses on climate change and has been instrumental in determining the crusade of the ozone hole over Antarctica. (credit: National Oceanic and Atmospheric Assistants)

Key Concepts and Summary

The ideal gas law can exist used to derive a number of convenient equations relating directly measured quantities to backdrop of involvement for gaseous substances and mixtures. Advisable rearrangement of the ideal gas equation may be made to allow the calculation of gas densities and molar masses. Dalton'south constabulary of partial pressures may be used to relate measured gas pressures for gaseous mixtures to their compositions. Avogadro'south police may be used in stoichiometric computations for chemical reactions involving gaseous reactants or products.

Key Equations

• [latex]P_{Full} = P_A + P_B + P_C + \cdots = \sum_\text{i} P_\text{i}[/latex]
• [latex]P_A = X_A P_{Total}[/latex]
• [latex]X_A = \frac{n_A}{n_{Total}}[/latex]

Chemistry Terminate of Chapter Exercises

1. What is the density of laughing gas, dinitrogen monoxide, N_twoO, at a temperature of 325 K and a pressure of 113.0 kPa?
2. Calculate the density of Freon 12, CF₂Cl₂, at 30.0 °C and 0.954 atm.
3. Which is denser at the same temperature and pressure level, dry out air or air saturated with h2o vapor? Explain.
4. A cylinder of O₂(m) used in breathing by emphysema patients has a book of 3.00 L at a pressure of 10.0 atm. If the temperature of the cylinder is 28.0 °C, what mass of oxygen is in the cylinder?
5. What is the tooth mass of a gas if 0.0494 g of the gas occupies a volume of 0.100 L at a temperature 26 °C and a pressure level of 307 torr?
6. What is the molar mass of a gas if 0.281 m of the gas occupies a book of 125 mL at a temperature 126 °C and a force per unit area of 777 torr?
7. How could you testify experimentally that the molecular formula of propene is C₃H₆, not CH_two?
8. The density of a certain gaseous fluoride of phosphorus is 3.93 chiliad/Fifty at STP. Calculate the molar mass of this fluoride and determine its molecular formula.
9. Consider this question: What is the molecular formula of a compound that contains 39% C, 45% Due north, and 16% H if 0.157 thousand of the chemical compound occupies l25 mL with a pressure of 99.5 kPa at 22 °C?

   (a) Outline the steps necessary to reply the question.

   (b) Answer the question.

10. A 36.0–L cylinder of a gas used for calibration of blood gas analyzers in medical laboratories contains 350 g CO_ii, 805 g O_two, and 4,880 g North₂. At 25 degrees C, what is the pressure level in the cylinder in atmospheres?
11. A cylinder of a gas mixture used for scale of blood gas analyzers in medical laboratories contains 5.0% CO₂, 12.0% O₂, and the residue N_ii at a total pressure of 146 atm. What is the fractional pressure of each component of this gas? (The percentages given indicate the percentage of the total pressure that is due to each component.)
12. A sample of gas isolated from unrefined petroleum contains 90.0% CH_iv, 8.nine% C₂H₆, and i.i% C₃H₈ at a total pressure of 307.2 kPa. What is the fractional pressure level of each component of this gas? (The percentages given indicate the percent of the total force per unit area that is due to each component.)
13. A mixture of 0.200 one thousand of H₂, 1.00 thou of N_ii, and 0.820 1000 of Ar is stored in a airtight container at STP. Notice the volume of the container, assuming that the gases exhibit platonic behavior.
14. About mixtures of hydrogen gas with oxygen gas are explosive. However, a mixture that contains less than iii.0 % O₂ is not. If enough O₂ is added to a cylinder of H_ii at 33.2 atm to bring the full pressure to 34.v atm, is the mixture explosive?
15. A commercial mercury vapor analyzer can observe, in air, concentrations of gaseous Hg atoms (which are poisonous) as low equally two × ten⁻⁶ mg/L of air. At this concentration, what is the fractional pressure of gaseous mercury if the atmospheric pressure is 733 torr at 26 °C?
16. A sample of carbon monoxide was nerveless over water at a total pressure of 756 torr and a temperature of 18 °C. What is the pressure of the carbon monoxide? (See Table two for the vapor pressure of water.)
17. In an experiment in a full general chemistry laboratory, a student nerveless a sample of a gas over water. The book of the gas was 265 mL at a pressure of 753 torr and a temperature of 27 °C. The mass of the gas was 0.472 g. What was the molar mass of the gas?
18. Joseph Priestley first prepared pure oxygen by heating mercuric oxide, HgO:
    [latex]ii \text{HgO}(s) \longrightarrow 2\text{Hg}(l) + \text{O}_2(thousand)[/latex]

    (a) Outline the steps necessary to answer the following question: What volume of O_ii at 23 °C and 0.975 atm is produced by the decomposition of 5.36 g of HgO?

    (b) Respond the question.

19. Cavendish prepared hydrogen in 1766 by the novel method of passing steam through a blood-red-hot gun barrel:
    [latex]4 \text{H}_2 \text{O}(g) + 3\text{Fe}(due south) \longrightarrow \text{Fe}_3 \text{O}_4 + four\text{H}_2(one thousand)[/latex]

    (a) Outline the steps necessary to answer the post-obit question: What volume of H₂ at a pressure of 745 torr and a temperature of 20 °C can be prepared from the reaction of 15.O g of H_twoO?

    (b) Respond the question.

20. The chlorofluorocarbon CCl₂F₂ tin can be recycled into a dissimilar compound by reaction with hydrogen to produce CH₂F_two(g), a compound useful in chemical manufacturing:
    [latex]\text{CCl}_2 \text{F}_2(thou) + 4 \text{H}_2(g) \longrightarrow \text{CH}_2 \text{F}_2(g) + 2\text{HCl}(g)[/latex]
    (a) Outline the steps necessary to answer the following question: What volume of hydrogen at 225 atm and 35.five °C would be required to react with 1 ton (one.000 × 10^three kg) of CCl₂F₂?

    (b) Respond the question.

21. Automobile air bags are inflated with nitrogen gas, which is formed by the decomposition of solid sodium azide (NaN₃). The other production is sodium metal. Calculate the volume of nitrogen gas at 27 °C and 756 torr formed past the decomposition of 125 thousand of sodium azide.
22. Lime, CaO, is produced by heating calcium carbonate, CaCO_iii; carbon dioxide is the other product.

    (a) Outline the steps necessary to answer the following question: What volume of carbon dioxide at 875° and 0.966 atm is produced by the decomposition of 1 ton (1.000 × x³ kg) of calcium carbonate?

    (b) Answer the question.

23. Earlier small batteries were bachelor, carbide lamps were used for wheel lights. Acetylene gas, C_iiH₂, and solid calcium hydroxide were formed by the reaction of calcium carbide, CaC₂, with water. The ignition of the acetylene gas provided the light. Currently, the same lamps are used by some cavers, and calcium carbide is used to produce acetylene for carbide cannons.

    (a) Outline the steps necessary to answer the following question: What volume of C₂H₂ at one.005 atm and 12.2 °C is formed by the reaction of 15.48 g of CaC₂ with water?

    (b) Respond the question.

24. Calculate the volume of oxygen required to burn down 12.00 L of ethane gas, C_iiH₆, to produce carbon dioxide and water, if the volumes of C_iiH₆ and O₂ are measured under the same conditions of temperature and force per unit area.
25. What volume of O_ii at STP is required to oxidize viii.0 L of NO at STP to NO₂? What volume of NO₂ is produced at STP?
26. Consider the post-obit questions:

    (a) What is the total volume of the CO₂(yard) and H₂O(thou) at 600 °C and 0.888 atm produced by the combustion of ane.00 L of C₂H_vi(k) measured at STP?

    (b) What is the fractional pressure of H₂O in the product gases?

27. Methanol, CH₃OH, is produced industrially by the following reaction:[latex]\text{CO}(k) + 2 \text{H}_2(chiliad) \xrightarrow{\;\;\;\;\;\;\text{copper catalyst} \;300 \;^{\circ} \text{C},\;300 \;\text{atm}\;\;\;\;\;\;} \text{CH}_3 \text{OH}(k)[/latex]
    Bold that the gases bear every bit platonic gases, notice the ratio of the full volume of the reactants to the final volume.
28. What volume of oxygen at 423.0 K and a force per unit area of 127.4 kPa is produced past the decomposition of 129.7 g of BaO₂ to BaO and O₂?
29. A 2.50-50 sample of a colorless gas at STP decomposed to requite 2.50 L of Northward₂ and 1.25 50 of O₂ at STP. What is the colorless gas?
30. Ethanol, C_iiH₅OH, is produced industrially from ethylene, C_iiH₄, past the following sequence of reactions:
    [latex]iii \text{C}_2 \text{H}_4 + 2\text{H}_2 \text{SO}_4 \longrightarrow \text{C}_2 \text{H}_5 \text{HSO}_4 + (\text{C}_2 \text{H}_5)_2 \text{SO}_4[/latex]
    [latex]\text{C}_2 \text{H}_5 \text{HSO}_4 + (\text{C}_2 \text{H}_5)_2 \text{SO}_4 + 3\text{H}_2 \text{O} \longrightarrow 3\text{C}_2 \text{H}_5 \text{OH} + two\text{H}_2 \text{And so}_4[/latex]
    What book of ethylene at STP is required to produce 1.000 metric ton (1000 kg) of ethanol if the overall yield of ethanol is 90.1%?
31. Ane molecule of hemoglobin will combine with four molecules of oxygen. If 1.0 thou of hemoglobin combines with ane.53 mL of oxygen at body temperature (37 °C) and a pressure of 743 torr, what is the molar mass of hemoglobin?
32. A sample of a compound of xenon and fluorine was confined in a bulb with a pressure level of 18 torr. Hydrogen was added to the bulb until the pressure was 72 torr. Passage of an electric spark through the mixture produced Xe and HF. Afterwards the HF was removed by reaction with solid KOH, the final pressure of xenon and unreacted hydrogen in the seedling was 36 torr. What is the empirical formula of the xenon fluoride in the original sample? (Note: Xenon fluorides comprise only one xenon cantlet per molecule.)
33. One method of analyzing amino acids is the van Slyke method. The characteristic amino groups (−NH₂) in protein material are allowed to react with nitrous acrid, HNO_two, to form N_two gas. From the volume of the gas, the amount of amino acid tin can exist adamant. A 0.0604-thousand sample of a biological sample containing glycine, CH₂(NH_two)COOH, was analyzed by the van Slyke method and yielded three.seventy mL of Due north₂ collected over water at a force per unit area of 735 torr and 29 °C. What was the percent of glycine in the sample?
    [latex]\text{CH}_2 \; (\text{NH}_2) \text{CO}_2 \text{H} + \text{HNO}_2 \longrightarrow \text{CH}_2 \;(\text{OH}) \text{CO}_2 \text{H} + \text{H}_2 \text{O} + \text{N}_2[/latex]

Glossary

Dalton's police of partial pressures

total force per unit area of a mixture of platonic gases is equal to the sum of the partial pressures of the component gases.

mole fraction (Ten)

concentration unit defined as the ratio of the molar amount of a mixture component to the full number of moles of all mixture components

partial pressure

pressure level exerted by an individual gas in a mixture

vapor pressure level of water

pressure exerted by h2o vapor in equilibrium with liquid water in a closed container at a specific temperature

Solutions

Answers to Chemical science End of Chapter Exercises

2. four.64 g Fifty⁻ⁱ

4. 38.viii g

6. 72.0 g mol⁻¹

viii. 88.1 g mol⁻¹; PF₃

10. 141 atm

12. CH_iv: 276 kPa; C₂H₆: 27 kPa; C_iiiH₈: 3.four kPa

14. Yep

xvi. 740 torr

18. (a) Determine the moles of HgO that decompose; using the chemical equation, determine the moles of O₂ produced past decomposition of this amount of HgO; and decide the book of O₂ from the moles of O₂, temperature, and pressure. (b) 0.308 Fifty

20. (a) Determine the molar mass of CCl₂F_ii. From the balanced equation, summate the moles of H₂ needed for the complete reaction. From the ideal gas law, convert moles of H₂ into volume. (b) 3.72 × x³ L

22. (a) Remainder the equation. Determine the grams of CO₂ produced and the number of moles. From the ideal gas law, determine the volume of gas. (b) 7.43 × 10⁵ 50

24. 42.00 Fifty

26. (a) 18.0 50; (b) 0.533 atm

28. 10.57 L O₂

xxx. 5.40 × 10⁵ L

32. XeF_two

---

mcanallyhimeaugh.blogspot.com

Source: https://opentextbc.ca/chemistry/chapter/9-3-stoichiometry-of-gaseous-substances-mixtures-and-reactions/

Share this post